Static indeterminacy and Kinematic indeterminacy: Refresh Structural Basic

Today, We will discuss about the concept about the degree of the Static indeterminacy and Kinematic indeterminacy. So let’s see-

Purpose of finding the degree of indeterminacy in structure:

We find the degree of indeterminacy to check whether the structure is stable or unstable. There no use of constructing any structure if it is not stable.

Types of the degree of indeterminacy:

1.The degree of static indeterminacy (Ds), 2.The degree of kinematic indeterminacy(Dk)

The Total indeterminacy of structure is given by the sum of the degree of static indeterminacy and kinematic indeterminacy. And for Static Indeterminacy-the total static indeterminacy is given by the sum of external static indeterminacy (DSe) and internal static indeterminacy (DSi).

The degree of Static Indeterminacy:

The degree of Static Indeterminacy is the number of Redundant Forces in Structure. Redundant Forces are the Forces that can not be determined by solving only the equations of Equilibrium. They are independent.

Therefore, the degree of Static Indeterminacy represent the difference between the number of static unknowns(Reactions & Internal Forces) and the number static Equations (Equilibrium Equations).

The degree of Kinematic Indeterminacy:

When a structure is loaded, it undergoes deflection or movement at the joints i.e. translation and rotation. If these unknown derivable(Translation & Rotation) can be found by applying compatibility conditions alone then the structure is Kinematically Determinate. However, if these unknown deflections cannot be found by compatibility conditions then the structure is Kinematically Indeterminate.

The Degree of Kinematic Indeterminacy is defined as the number of non-zero joints displacement of the structure. It is also called the Degree of freedom (DOF).

Formula Chart for Degree of Indeterminacy:

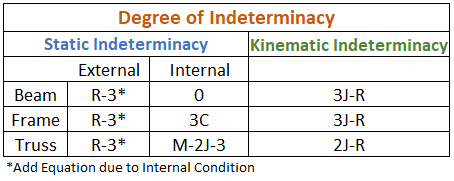
Where, R=Number of unknown Reaction, C=Number of Closed Loop, M=Number of Member, J=Number of Joints.

For basic knowledge to calculate degree if kinematic indeterminacy let us read the chart below:

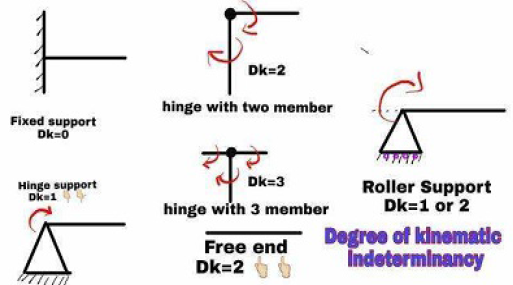
If there is a fixed end then there will not be any value of kinematic indeterminacy and tends to zero. In hinged support, dynamic indeterminacy will be one (1).
In roller support, kinematic indeterminacy will be two (2) with axial deformation and one (1) if axial deformation is neglected. If there is an internal hinge with two members then dynamic indeterminacy will be two(2).
If the internal hinge is with three members then dynamic indeterminacy will be three (3).
For the free end, the dynamic indeterminacy will be two (2).

Example:

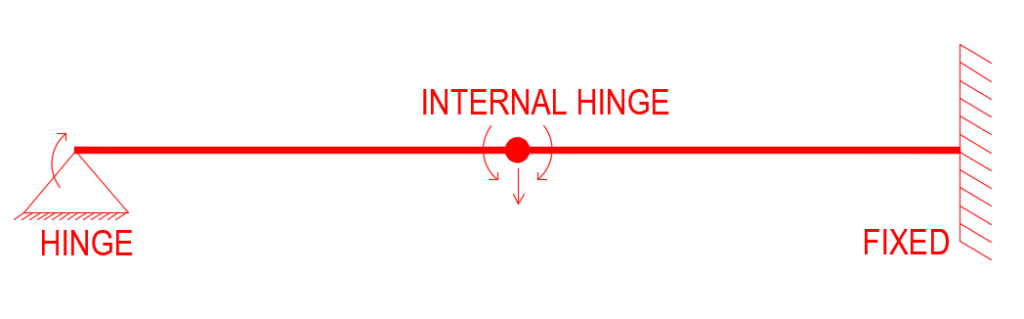
We have the Equation, Ds = DSe + DSi——————(1)

Where, Ds= Degree of Static Indeterminacy, DSe=Degree of External Indeterminacy, DSi=Degree of Internal Indeterminacy.

DSe=R-3-(Equation for Internal Hinge Condition)=R-3-(M-1)

[If there is 2 internal hinge then the Equation will be R-3-(M-1)-(M-1), similarly for each Internal Joint there will be additional equation of M-1]

Here, Unknown Reaction in two support condition, R=2+3=5, Number of Member connected with the internal Hinge, M=2.

So, DSe= 5-3-(2-1)=1

And From Chart DSi=0 for Beam.

So, Degree of Static Indet. Ds= 1+0=1

For Kinematic Indeterminacy:

At 1st Joint(hinge) Dk=1, At 2nd Joint (Int. Hinge) Dk=3, And at last Joint Dk=0

So, for the structure shown, Dk=1+3+0=4